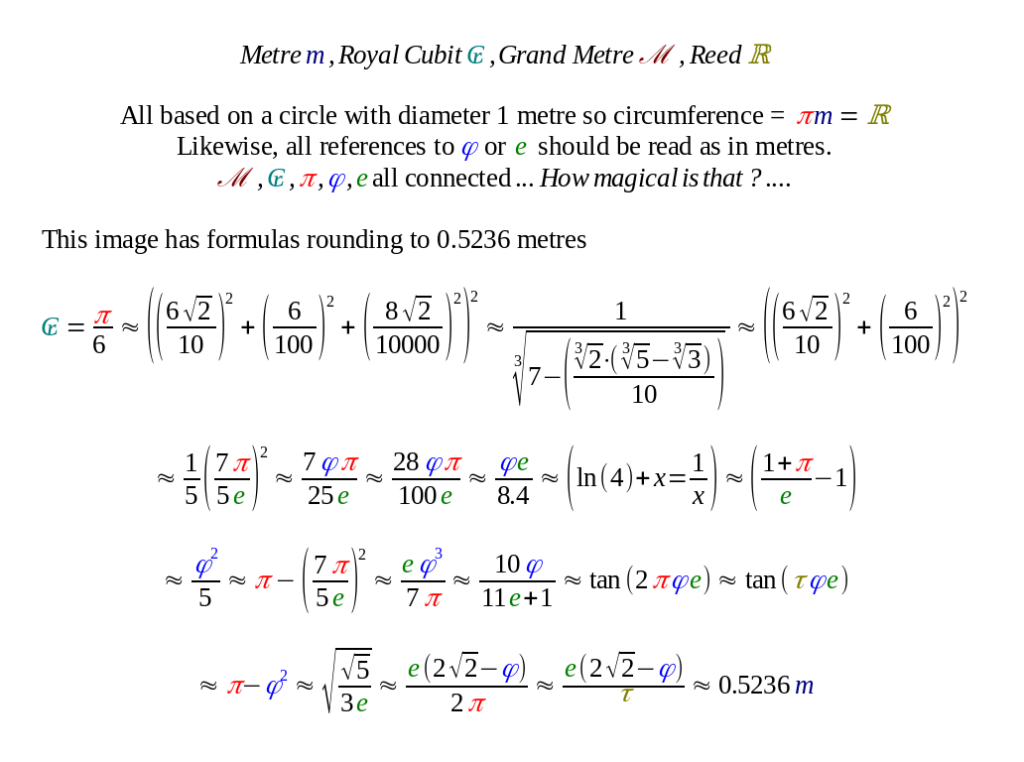
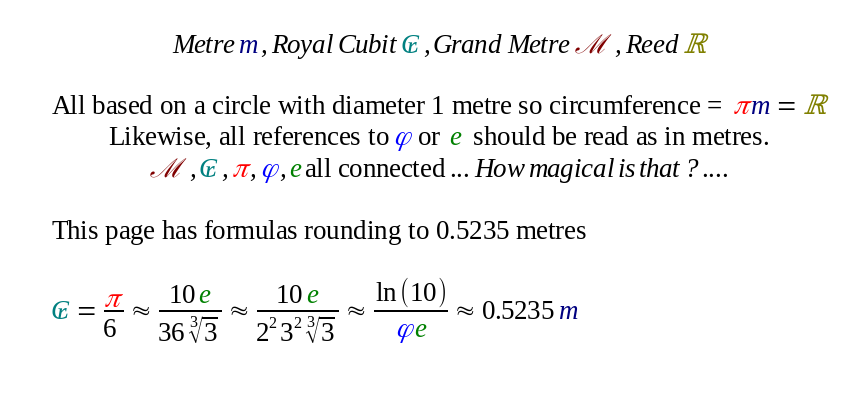
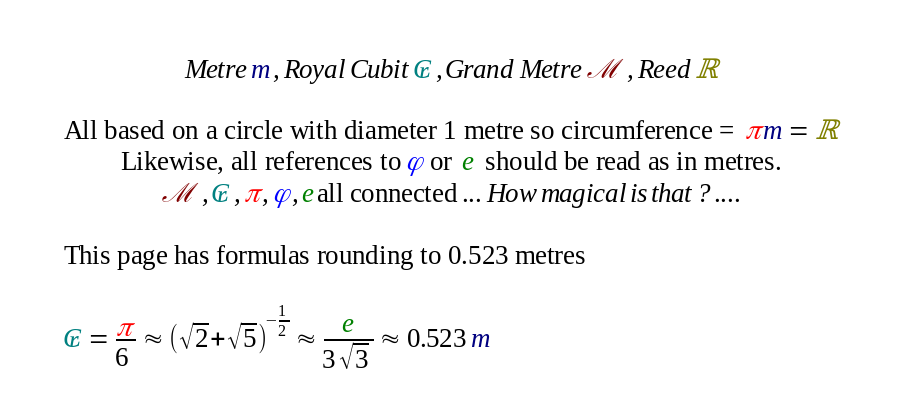
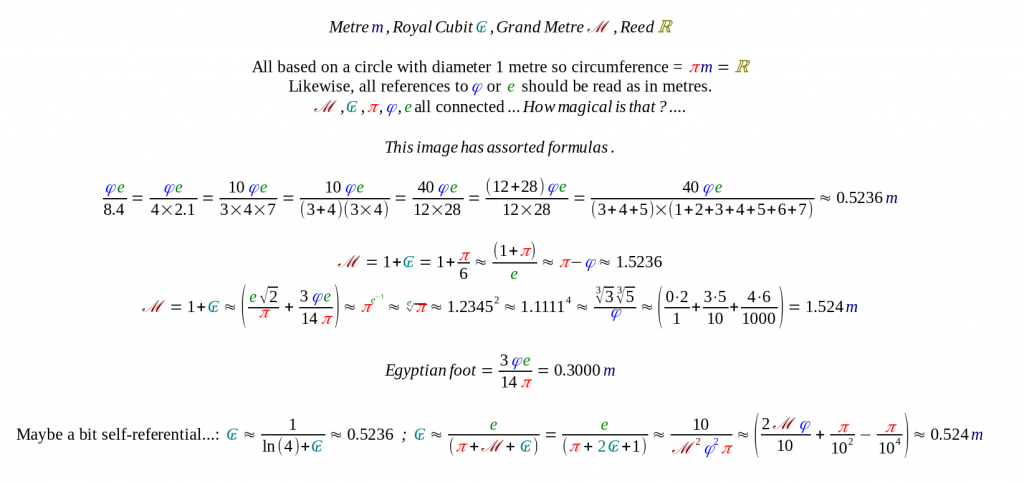
Let the maths do the talking….

Click images to enlarge.

Cubit formulas rounding to 0.5236 m

Formulas rounding to 0.5235m

Cubit formulas rounding to 0.524 m

Cubit formulas rounding to 0.523 m

Other cubit formulas

I’m leaving out 1/√(364.75/100) until such time as I have a better explanation for it. (Although it does effectively pop up in the discussion about the nautical mile.)

According to Wikipedia, the Reed was different things at different times … at one point being only 2 cubits, about 1 metre, long. They also called the 6-royal-cubit length “kalamos” which is called the Reed in the image above. Given that we’re dealing with civilizations that lasted thousands of years, it’s hard to know what’s what.

I suspect that’s why there are different versions of the Royal cubit floating around … on the one hand, copy errors, on the other people using actual body parts to create measurements. It is my humble opinion that whoever came up with these things did it very logically, based on maths, and the metre and pi and phi. The big remaining question is how the heck did they get the metre.

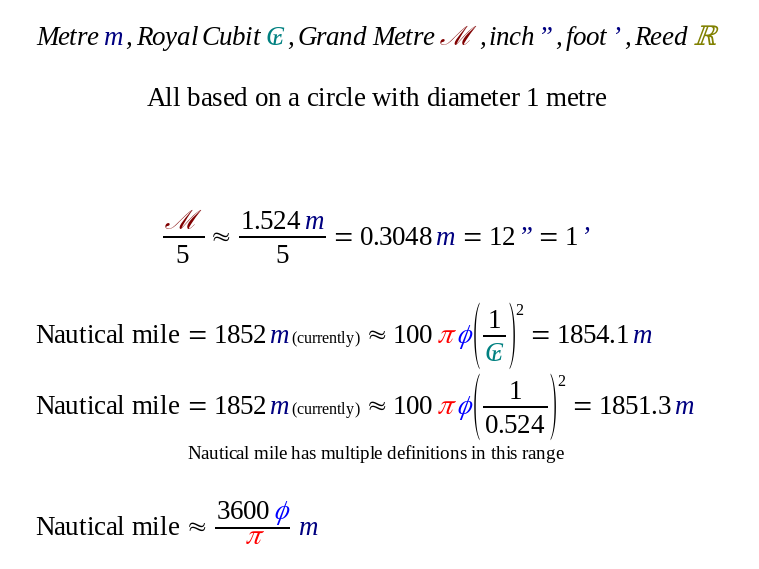
And in relation to the foot, inch and nautical mile:

Cubit and imperials.

[Note: this image has been updated a few times as I stumble across alternate formulas, should have added a changelog. Latest updates:
2018-11-24: added the cuberoot(5)φ²/πe]
2018-11-26: added (e φ³)/(7 pi)
2018-11-27: added (e√2/π) + 3φe/14π
2018-11-27: added e(2√2 – φ)/2π
2018-11-28: added (φe)/8.4, (10φ)/(11e + 1), and (φ)/(e + 1/e). Found out how to get proper φ in LibreOffice Maths. Added 1/(√(ln365.25/φ)).
2018-12-02: added 28φπ/100e
2018-12-03: Added formulas based on rewrites of the 5φe/7π == 1 formula, rearranged the Royal Cubit approximations to split more exact from less exact.
2018-12-04: Added pi^(1/e) to Grand Metre section, rearranged that section a bit, plus other tweaks.
2018-12-08: Added 2√5 / φe and 10√2 / 3³
2018-12-10: Added formula based on ln(4)
2018-12-12: Added 1/log(φ²π³), sin(φ²πe√2)
2018-12-13: Added tan(2φπe), cos(π(4φe+1))
2018-12-14: Added e/3√3, some other rearranging.
2018-12-13: Added √(√5/3e), a better formula using just e. Found by serendipity.
2018-12-18: Added π/(φ³√2)
2019-01-15: Split image into smaller parts, added formulas based on multiples of √2, added tau equivalents to some pi formulas
2019-02-10: Added formulas rounding to 0.5235m which somehow got dropped.