Some more fake news magazine covers, from December 2019.
Douglas Modern Chess, Season 2 Heat 1
Herewith the results from heat 1. Time control was 5 minutes plus 10 seconds per move.
Summary:
Games: 12; Draws: 4, DrawPercentage: 33.3%
Whitewins: 5; Blackwins: 3, Draws: 4
Conventional scoring:
Raubfisch_ME262_GTZ20d3._sl : 5.5 Zeus 4.1.7 M : 4 rofChade 2.203 : 1.5 Komodo 11 : 1
Results table:
Engine Win Draw Lose Raubfisch_ME262_GTZ2 5 [3/2] 1 [0/1] 0 [0/0] Zeus 4.1.7 M 3 [2/1] 2 [1/1] 1 [0/1] rofChade 2.203 0 [0/0] 3 [2/1] 3 [1/2] Komodo 11 0 [0/0] 2 [1/1] 4 [2/2]
My scoring system which takes black/white into account:
Raubfisch_ME262_GTZ20d3._sl : 16.46 Zeus 4.1.7 M : 10.18 rofChade 2.203 : -2.9 Komodo 11 : -9.89
My points-based scoring system, which takes black/white and number of moves into account:
Engine Points Percentage Raubfisch_ME262_GTZ20d3._sl : 274 : 91.33% Zeus 4.1.7 M : 198 : 66% rofChade 2.203 : 74 : 24.67% Komodo 11 : 50 : 16.67%
Cutechess scoring:
Rank Name Elo +/- Games Score Draws 1 Raubfisch_ME262_GTZ20d3._sl 417 nan 6 91.7% 16.7% 2 Zeus 4.1.7 M 120 333 6 66.7% 33.3% 3 rofChade 2.203 -191 238 6 25.0% 50.0% 4 Komodo 11 -280 nan 6 16.7% 33.3%
So Raubfisch_ME262_GTZ20d3._sl and Zeus 4.1.7 M go through to the semi-finals.
Herewith the games. Mate of the Match award goes to the Raubfisch_ME262_GTZ20d3._sl vs Zeus 4.1.7 M game, towards the bottom.
Douglas Modern Chess, Season 2
Now that Season 18 is mostly done over at https://tcec-chess.com/, I thought I’d run another round of the Modern Chess, using updated versions of the engines.
I did try to add LC0 and SugarNN, but could not get them to function properly. It may be because my GPU is rather old, or some back-end dependency was not available.
I did some preliminary testing, to give some newcomers a chance to qualify. The following engines did not survive. Ethereal was a surprise, given how well it did compared to Fire on TCEC.
Ethereal 12
Defenchess 2.3 dev
Godel 7.0
Raven 0.8
zct-032500-64-ja
The following engines did make the cut. In essence it’s a bit of a Stockfish bloodfest, since apart from Stockfish itself, there are three derivatives.
Stockfish 11 (not the latest available, but latest official release)
Raubfisch_ME262_GTZ20d3._sl (based on Stockfish)
Raubfisch X41d3._sl (based on Stockfish)
Zeus 4.1.7 M (based on Stockfish)
Komodo 11
xiphos-0.6-linux-sse (not updated since Season 1)
Fire_7.1_x64 (not updated since Season 1)
rofChade 2.203
The results from Heat 1 will follow.
Fake News part 1
There has been a lot of fuss in recent years about the so-called “fake news,” as if this is a new development. Governments and religions have been blatantly lying since forever, and many things written as fiction somehow got accepted as “truth.”
Once the printing presses arrived, the problem exploded exponentially, and, since it is not illegal to publish lies, publishers had a field day, conveniently claiming their “news” was “entertainment” when challenged. So Elvis swims with the Loch Ness monster.
Anyway, the supermarket checkout aisles are stacked with examples of “fake news” which is never condemned, so this is a series of such covers. They’re not current, lest someone accuse me of spreading fake news.
Approximating the Fine Structure Constant (again) aka There’s Something About φ
While poking around Giza, I stumbled on this approximation for the inverse of the fine structure constant. It’s not as good as my previous one, but since it relies on cubit -> metre conversion, it qualifies as “interesting.”
It’s just 100φ² ₢, expressed as metres, So we just multiply that by π/6, and voila!
137.0799391
Which according to Wolfram Alpha,
100 / (100φ²π/6) / (fine structure constant)
is 99.9679457% accurate …
Well, according to the current known value, which is a moving target.
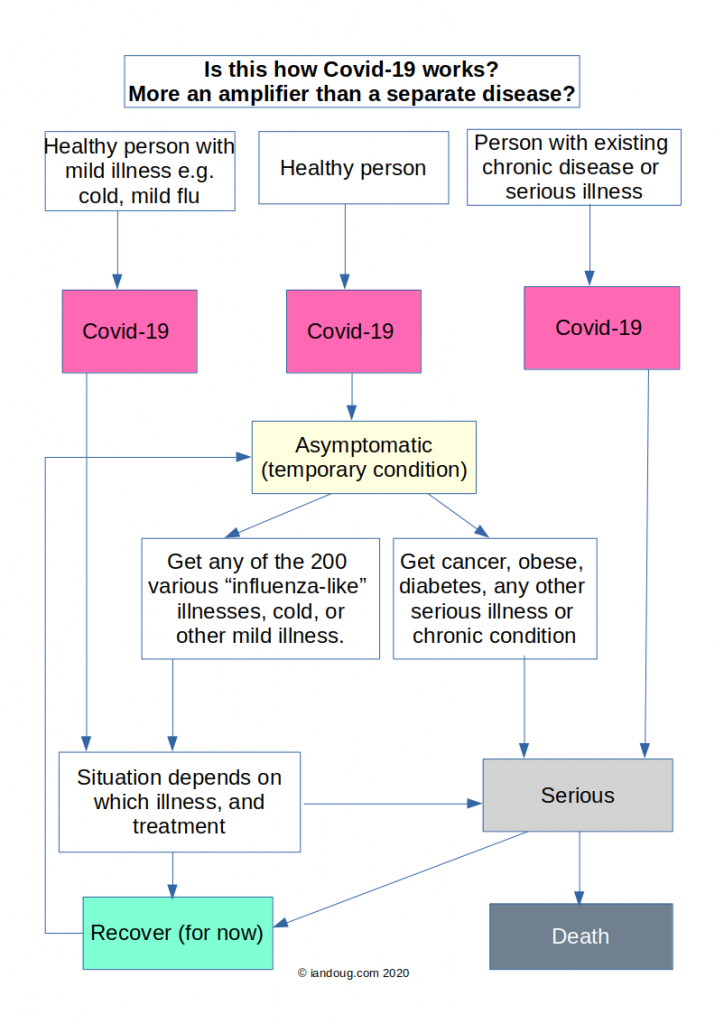
Covid-19 decoded
I’ve been tracking Covid-19 since January, and even have an analysis site at https://yo.co.za. Based on all the information available to date, this image shows how I think the disease actually works … it’s not just another type of influenza, but an accelerator of multiplier of whatever illness you have or get.
Disclaimer: I am not a doctor or medical professional, this is just my understanding of how it actually works.
Approximating the Fine Structure Constant
The fine structure constant is described as one of the most fundamental physical constants. I don’t pretend to understand anything about it apart from its value, which is close to 1/137. We’ve only been using it for around 100 years.
So the fact that the Khafre pyramid uses 137 as a size multiplier (base is 3 x 137, height is 2 x 137) is annoying, especially since the Khufu pyramid references the speed of light in metres per second, twice. The ancient Egyptians are not supposed to know those things.
Since the fine structure constant itself is a messy decimal number (0.0072973525693…) it is usually referenced via its inverse, as 1/137, which provides a reasonable approximation.
However there have been attempts to find a better approximation, and Scott Onstott has a page with some approximations. Which naturally was a challenge I could not resist….
So I saw that one of the better ones was based on 137²+π²…. and since the royal cubit is π/6, and I have lots of approximations for that, it was simply a matter of finding the right one that produced a better result. That turned out to be based on the plastic number… so without further fanfare, here we are:
\frac{1}{\alpha} \approx \sqrt{{137^{2} + (\frac{\rho^{9}}{4}})^{2}}
The WolframAlpha version of the formula is √(137² + ((plastic number^9)/4)²), which produces
137.0359992970725102075551820936909082242952278384186387671
We can check the percentage accuracy as well:
100/137.0359992970725102075551820936909082242952278384186387671 / (fine structure constant)
produces 99.9999998% accurate. Not bad.
The Secret is as Simple as e
I updated my paper on the alignment of the pyramids again, and included some new diagrams. The most important of which is this stunning bit of simplicity. As a reminder. ⦦e means “360/e”, which is 132.437°. The pure elegance of this may be disruptive to existing theories about the alignment of the pyramids. You read it here first 🙂 (Always wanted to say that.)
You can see the paper itself for the accuracy table. Two are accurate to less than 0.1° and the other is below 0.3°.
408, 199 and 159
A follow-up to the 437 post, this time applying the same methodology to Khafre and the other two pyramids.
If you have seen my papers then you will know that Khafre is often connected to the number 3. This same idea pops up here.
So …. Khafre has a base length of 411 ₢. 411 – 3 is 408.
If we had a square with side 408, then the diagonal would be 408√2, or 576.999.
If we divide 1000 by that (a process which effectively takes the inverse, and fixes the location of the decimal point), then we get
1000/576.999 = 1.733104858, which is a close approximation of √3 … it differs by 0.0010540… Accuracy is of course limited by having to start with whole-cubit dimensions.
I have tried the same approach with the other two pyramids, as follows. SInce we are using smaller dimensions to start with, accuracy does suffer a bit:
437
While playing around with the calculator, looking at relationships between the numbers at Giza, I took another look at the diagonal of the great pyramid.
The great pyramid has a base of 440 ₢. This means the diagonal is 440√2, which is 622.25 ₢. This is about 4 ₢ more than 1000/φ, which is 618 ₢. The error percentage is about 0.6%, which is annoyingly close.
So I got to wondering what base size would produce a diagonal more or less exactly 1000/φ.
The answer turns out to be 437, which is 3 ₢ less the existing size.
437√2 = 618.011 which is as close as you are going to get using whole-cubit dimensions.
But the oddness does not stop there.